F/Maths OBJ:
1BCADDDDDBB
11DBABCCDDCC
21BCABBAABAB
31CBADBDDCAB

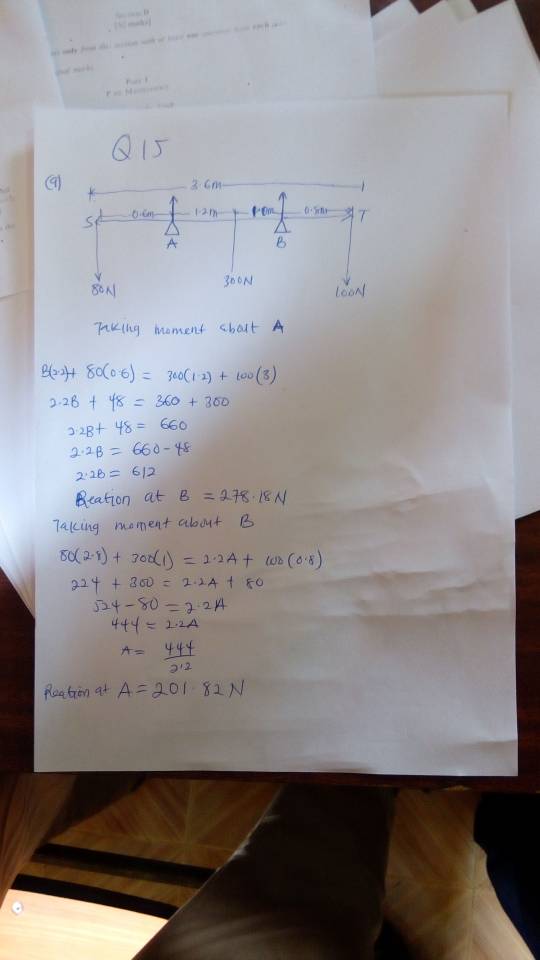
15a)
(2.2) + 80(0.6) = 300(1.2) + 100 (3)
2.2B + 48 = 360 + 300
2.B + 48 = 660
2.2B = 660 – 48
2.2B = 612
Reation at B = 278.18N
Taking moment about B
80(2.1) + 300(1) = 2.2A + 100 (0.8)
224 + 300 = 2.3A + 80
524 – 80 = 2.2A
A = 444/2.2
Raction a+ A = 201.82N

15b)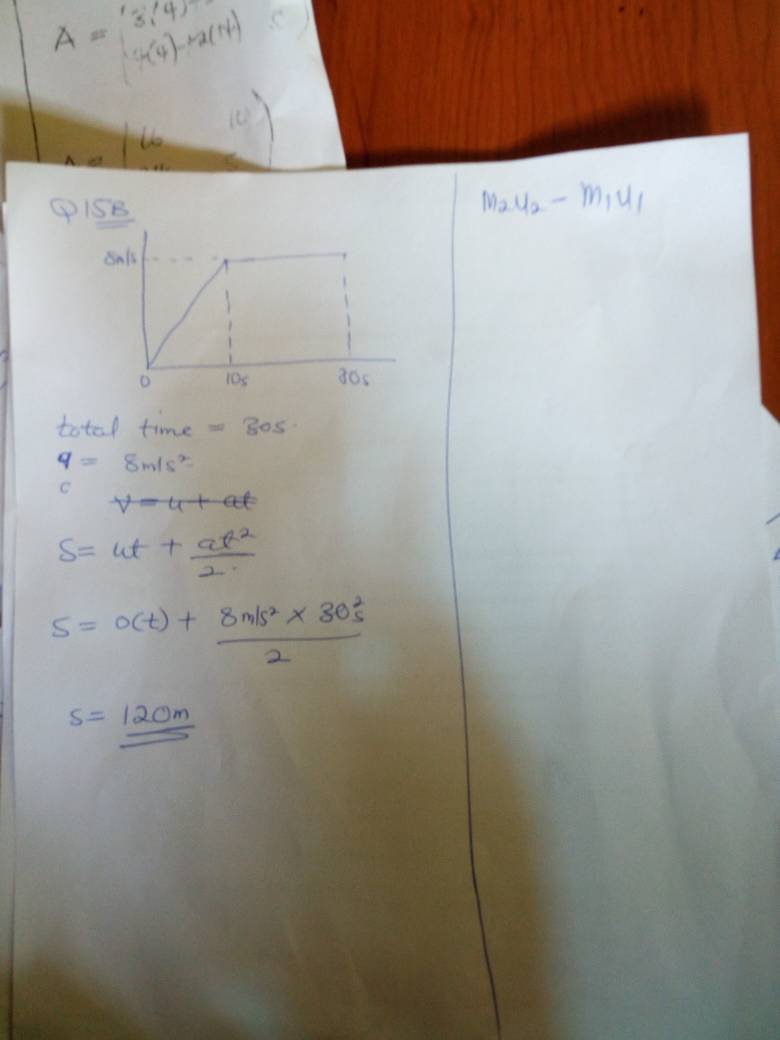

12)
TABULATE
No of head|x 0|12|3|45|6|7|8|9|10
Frequency|f|2|7|23|36|11|61|100|12|8|5|3
Fx| 0|7|46|108|44|305|600|84|64|45|30
Ef = 268 Efx = 1333
(a) mean no heads = Efx/Ef = 1333/268 = 4.97
(b) prob (even no heads) = 23/268 + 11/268 + 100/268 + 8/268 + 3/268
= 145/268
(c) prob (odd as heads) 7/268 + 26/268 + 61/268 + 12/268 + 5/268
= 121/268

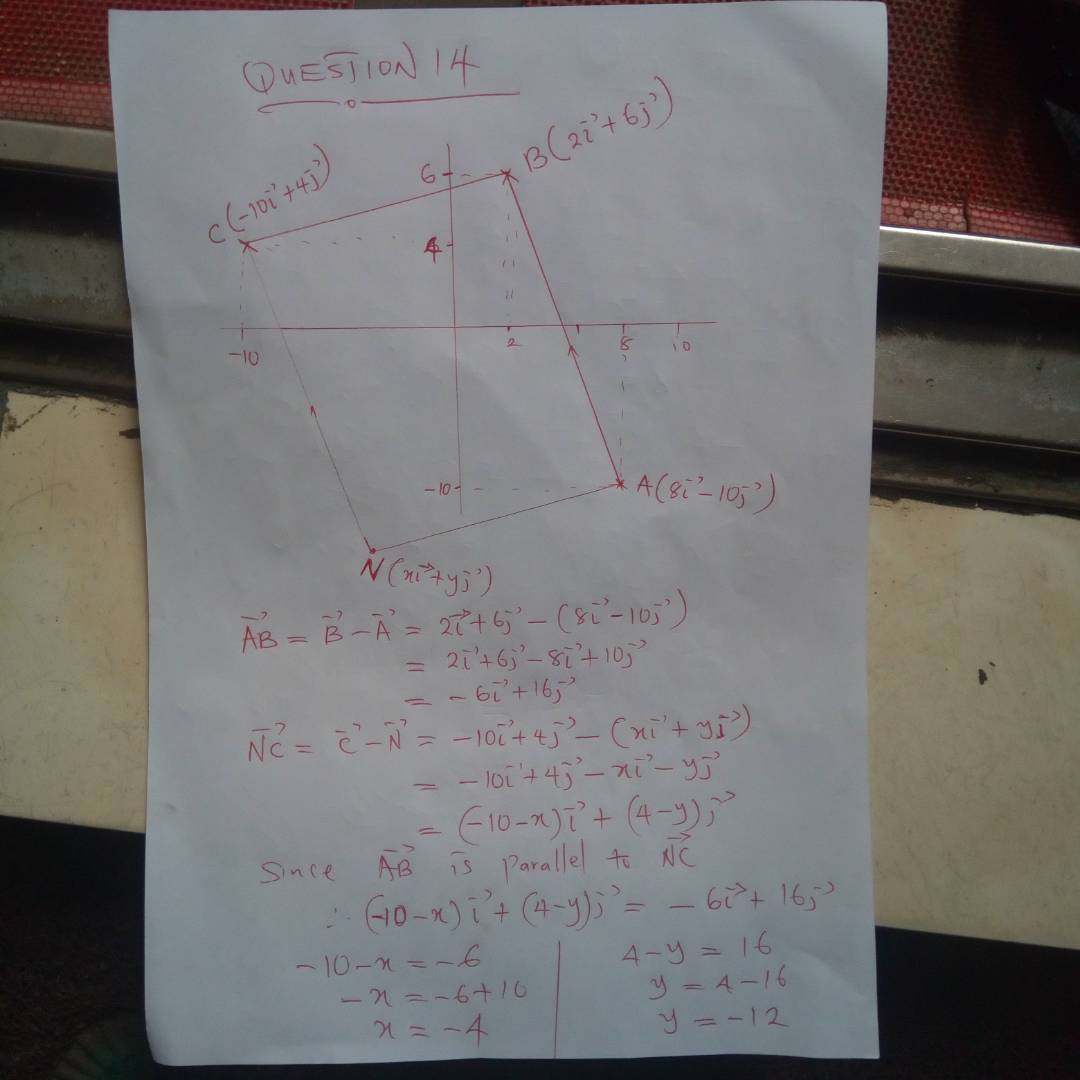
14)

9)
Draw the pie chart diagram
radius is the same hence
(y – 2)² + (X – 3)² = (y + 4)² + (X – 5)² = (y + 1)² + (X + 2)²
Taking the first pair
Y² – 4y + 4 + x² – 6x + 9 = y² + 8y + 16 + x² – 10x + 25
– 6x – 4y + 13 = -10x+8y+41
4x – 12y = 28
X = 3y = 7——–(1)
Taking the second pair
Y² + 3y + 16+x² – 10x+25 = y²+ 2y + 1 + x²+ 4x + 4
-10x + 3y + 41 = 4x + 2y + 5
14x – 6y = 36
7x – 3y = 18———(2)
Eqn 2 minus Eqn 1
6x = 11
X = 11/6
Put this into Eqn (1)
11/6 – 3y = 7
3y = 11/6 – 7
3y = 11 – 42/6
3y = -31/6
y = -31/18
(a) coordinates of centre is (11/6, -31/18)
(b) Radius r = √(y-2)²+(x-3)²
r = √-31/18 -2)² + 11/6 – 3)²
r= √13.85 + 1.36
r = 3.9
(c) (X – 11/6)² + (y + 31/18)² = 3.9²
(X – 11/16)² + (y + 31/18)² = 15.21

2)
Log 3x – 3logx³+ 2 = 0
Log 3x – 3log3³/log3x + 2 = 0
Log3x – 3/log3x + 2 = 0
P – 3/p + 2 = 0 where P = log3x
P² – 3 + 2p = 0
P² + 2p – 3 = 0
(p + 3) ( p – 1) = 0
P = -3 OR p = 1
But log3x = p
when p = -3
Log3x = -3
X = 3-³ = 1/27
And when P = 1
Log3x = 1
X = 3¹ = 3
And X = 1/27 OR 3

3a)
U=x-2 hence x=u+2
therefore
x^2+5/(x-2)^4
=(u+2)^3+5/(u+2-2)^2
=(u+2)^3+5/u^4
3b)
(u+2)^3+5/u^4
=(u+2)^3/u^4+5/u^4

4)
sn=n/2[2a+(n-1)d]
s12=12/2[2a+11d]
s12=6(2a+11d]=168
2a+11d=28 eq—–(1)
multipply 1by2 to obtain
2a+4d=14 eq——(3)
subtract (3)from(2)
7d=14
d=2
sub for d in (1)
a+4=7
a+7-4-3
a=3
common difference d=2
first term a=3

5)
tabulate
X,y,d,d^2
A 8,6,2,4
B 5,3,2,4
C 1,4,-3,9
D 7,8,-1,1
E 2,5,-3,9
F 6,7,-1,1
G 3,1,2,4
H 4,2,2,4
Ed^2 =4+4+9+1+9+1+4+4
=36
r=1-6£d^2/n(n^2-1)
=1-6*36/8(8^2-1)=1-216/504
=1-0.43
=0.57

1)
X-3(12-2x+8)+4(30-5x-4)+3(-20-4)=24
X-3(20-2x)+4(26-5x)+3(-24)= -24
20x-2x^2+60+6z+104-20x-72=24
20x^2+6x-60+104-72+24=0
-2x^2+6x-4=0
Divide 2x by -2
x2^2-3x+2=0
x2^2-2x-x+2=0
x(x-2)-1(x-2)-0
(x-1)(x-2)=0
x-1 or x=2

6)
It follow that:
P(n) = 1/3 and p(k)¹ = 1 – 1/3 = 2/3
P(T) = 1/5 and P(T)¹ = 1- 1/5 = 4/5
Hence, probability that only one if the them will be solve the questions will be:
= (1/3 × 4/5) + (1/5 × 2/3)
= 4/15 + 2/15
= 6/15
= 2/5

8a)
m1 = 20kg
u1 = 8ms-1
m2 = 30kg
u2 = 50ms-1
(a) in same direction
m1u1 + m2u2 = (m1+m2)v
20 × 80 + 30 × 50 = (20+30)v
1600+1500 = 50v
3100/50 = 50/50
V = 62ms-1
8b)
M1=20kg
u1=80mls
m2=30kg
u2=50mls
v=?
M1u1+m2u2=v(m1+m2)
(20*80)+(30*(-50))=v(20+30)
1600-1500=50v
100=50v
v=100/50
v=2mls

7)
m = 3i – 2j ; n = 2i + 3j ; p = i + 6j
Therefore:
4(3i – 2j) +2(2i +3j) -3(-i + 6j)
12i – 8j + 4i + 6j + 3i – 18j
12i + 4i + 3i – 8j + 6j – 18j
19i = 20j

GOOD LUCK