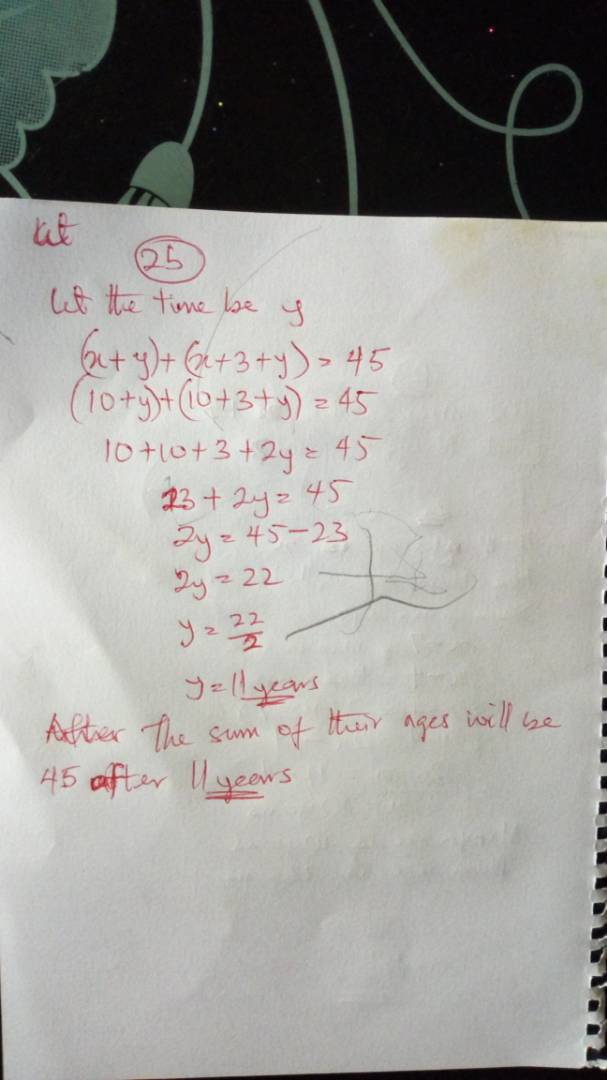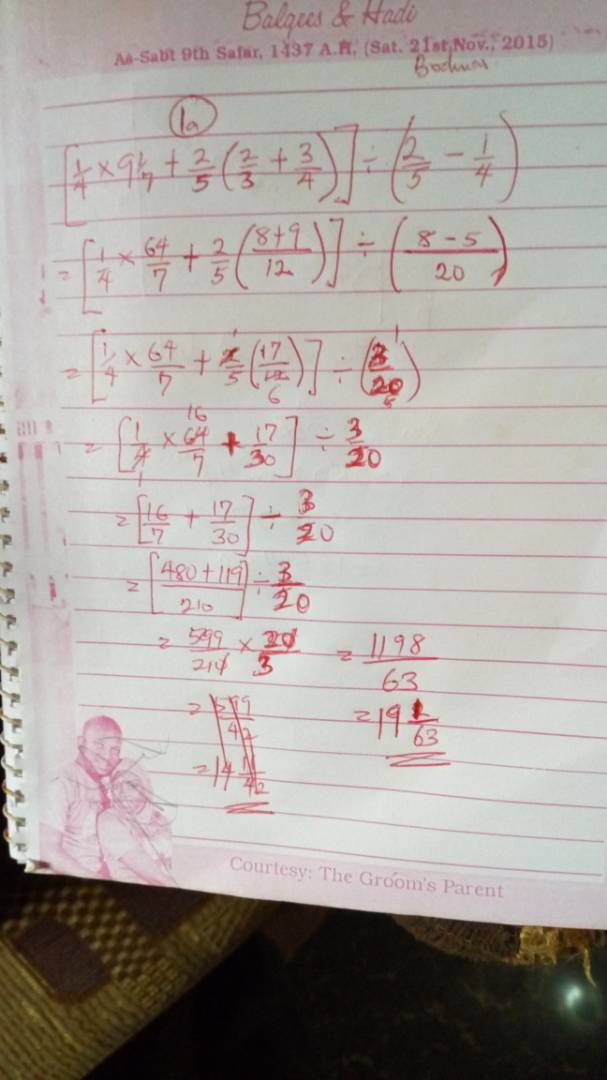Maths Obj

1 ACCAACDBBB

11BCABABCABB

21DCCCBCBCCA

31BDADBBDBAA

41DABCDBADAC

completed

Maths – Theory- Answers

Please anyone you didn’t understand kindly scroll down to see where it was solved on paper for clear understanding.

(11a)

Loga(y + 2) =  1 + LogaX

=> Log^y a +  Log^2 a = Log^a a + Log^x a

Loga^(y + 2) = Loga^(ax)

Y + 2 = ax

Hence y+2/a =  ax/a

X = y+2/a

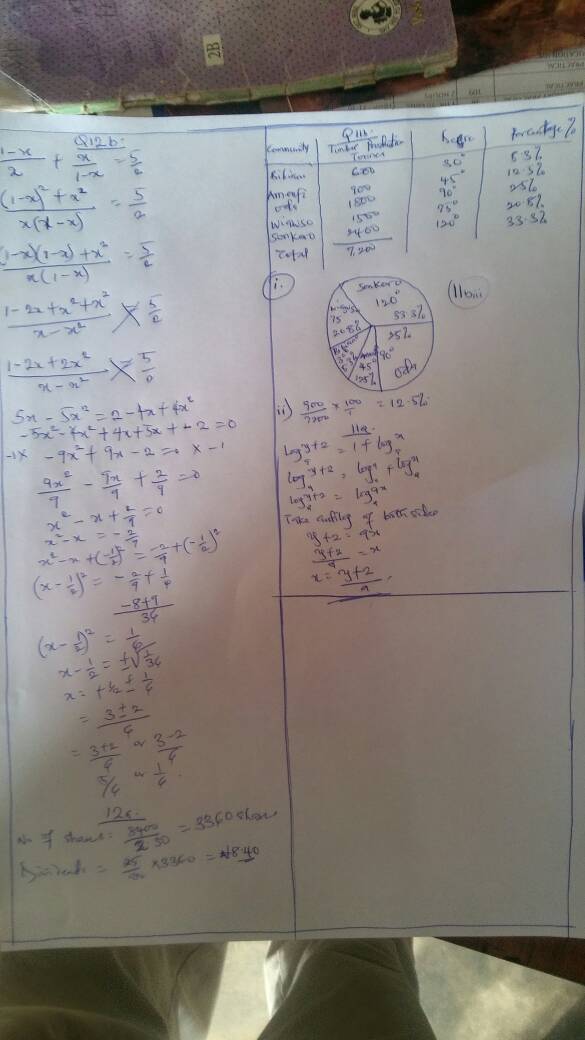
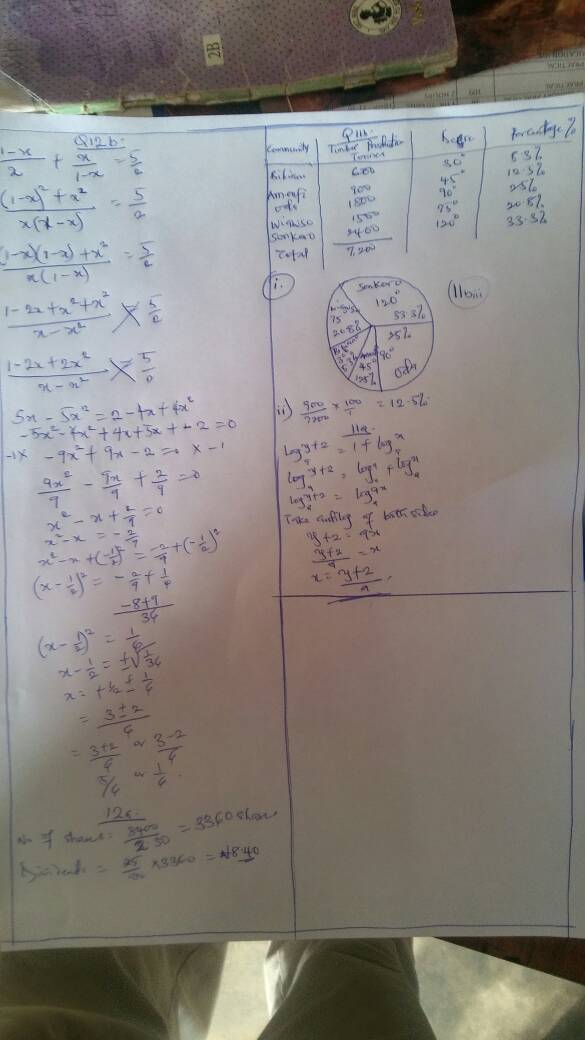
(11bi)

Bibiani =  600

Amenji = 700

Oda = 1800

Wawso = 1500

Sankose=2400

Total =     7200

Bibiani  = 600/7200 × 360/1 = 30°

Amenji  = 700/7200 × 360/1 = 45°

Oda = 1800/7200× 360/1 = 90°

Wawso = 1500/7200× 360 = 75°

Sankose = 2400/7200× 360/1 = 120°

Total = 30°+45°+90°+75°+120° = 360°

(11bii)

% of timber produced from Amenji = 900/7200 × 100/1 = 12.5%

(11biii)

Revenue received by Bibiani = 600×$560 = $336,000

Revenue received by Oda = 1800×560 = $1,008,000

Oda will receive(1,008,000 – 336000) = $672,000 more than Bibiani

( 4 a)

Rate = 2 / 100 * N 0 . 02 per month

Rate per annum = 0 . 02 * 12 = 0 . 24 per annum

( 4 b ) Draw the Diagram

< ZWY = < XWY = 180 degree( opp angles of cyclic quad . are supplimantary )

< ZWY = 100 = 180

< ZWY = 180 – 100

< ZWY = 100 degree

( 3 a)

[ Diagram ]

Distance covered by an athlete = Perimeter of A + Perimeter of rectangle CDEF + perimeter of B

Perimeter of A = 2 ?r / 2 = ? =22 / 7 , r = d / 2 = 120 / 2 = 60m

= 22 / 7 × 60 = 1320 / 7 = 188 . 57 m

Perimeter of B = perimeter of A = 188 . 57m

Perimeter of rectangle CDEF = 2 ( L + B )

L = 120 m ; B = 60m

Perimeter = 2 ( 120 + 60) = 2 ( 180 )

=360 m

Distance covered by an athlete = 188 . 57 + 360 + 188 . 57

=737 . 14 m

If the athlete runs the track two times = 2 × 737 . 14

= 1474 . 28m

( 3 b )

If the athlete spends 200 seconds for the race

Speed = distance / time

Distance = 1474 . 28 m

Time = 200 second

Distance = 1474 . 28 m = 1 . 47428km

Time = 200 seconds = 3 . 3333 hrs

Speed = 1 . 47428/ 3 . 3333 = 0 . 44kmhr – 1

( 6 a)

Tanx = 5 / 12

Using the diagram

Sinx = 5 / 13

Cosx = 12/ 13

Sinx / ( sinx ) ² + cosx = 5 / 13 / ( 5 / 13 ) ² + 12 / 13

= 5 / 13all over 25/ 169 + 12/ 13

= 5 / 13/ 25+ 156 / 169

=5 / 13 / 181 / 169

= 5 / 13 × 169 / 181 = 65/ 18

( 6 b )

9 b )

( PR ) ² =( PS ) ²+ ( SR ) ²

( PR ) ² =15² + 15²

( PR ) ² =225 + 225

( PR ) ² =450

PR =sqr root 225 ×2

PR =15 root 2 cm

But OR= PR ÷ 2 = 15root 2 ÷ 2

=7 . 5 ×1 . 4142

=10 . 6065

7 a)

Reduction in the first sales = 40 %

Reduction in the second sales = 30 %

Price sold Ghc 3500 = 70% ie ( 100 – 30) %

GHc y = 100 % second reduction sale

35 × 100 = 70y

35 × 100 / 70 = 70/ 70

Y = 350 / 7 = 50

Hence price after first sale = GHc50

But GHc 50 = 60% ie ( 100 – 40 ) %

Therefore GHcx = 100 % first reduction sale

100 × 50/ 60 = 60x / 60

X => 500 / 60 = GHc83 . 33

=>GHc 83. 3

Hence price before the first sales = GHc83 . 33

7 b )

Initil price of article = GHc = 180 . 00

In the first sales, reduction = 40 %

i . e 100 % – GHc 18 . 00

40 % – GHc x

100 x / 100 = 40 * 180 / 100

. : . x = 4 * 18 = GHc 72 . 00

Since reduction in the first sale is GHc 72 . 00

Then reduction in the second = 30 %

100 % = GHc 108

30 % = y

100 y / 100 = 30 * 108 / 100 = 324 / 10 = GHc 32 . 4

( i ) Hence reduction in the price due to the two sales = ( 72 + 32 . 4 ) GHc = GHc 104 . 4

( ii ) % reduction = Reduction / Original price * 100 / 1

=104 . 4 / 180 * 100 / 1 = 58 %

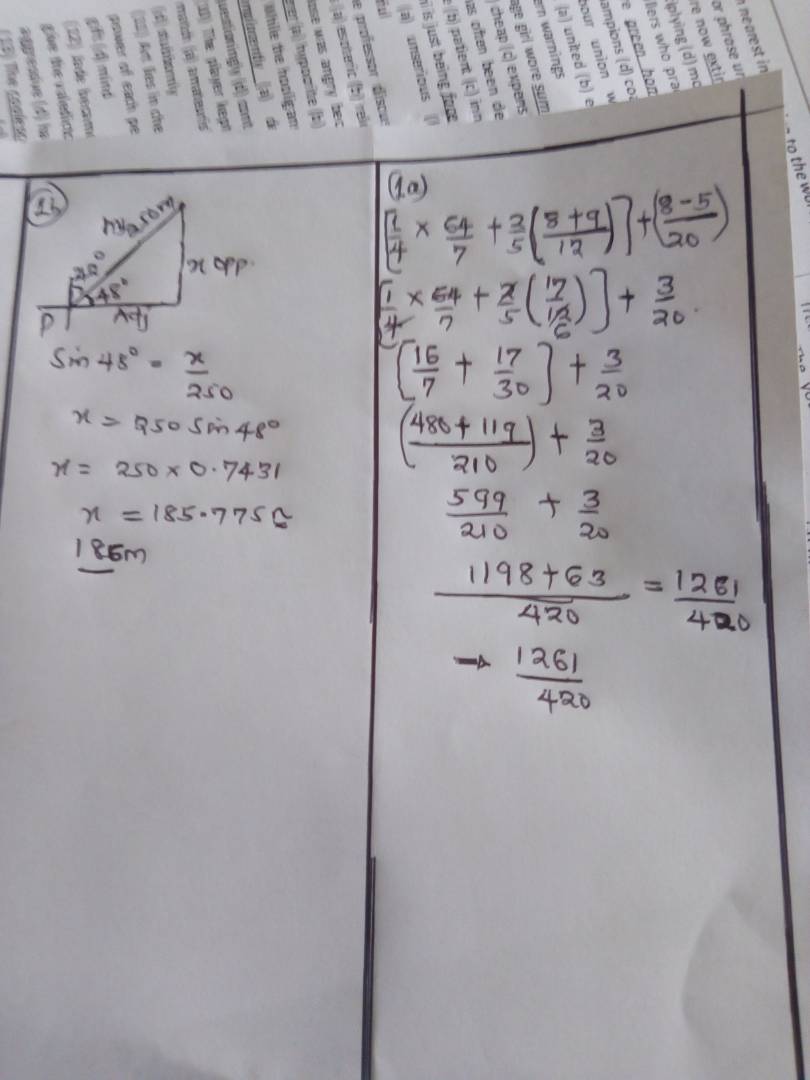
1 )

1 / 4 * 9 1 / 7 + 2 / 5 [ 2 / 3 + 3 / 4 ] / ( 2 / 5 – 1 / 4 )

( 1 / 4 * 64 / 7 + 2 / 5 ) [ 17 / 12)] / 8 – 20 / 20 ]

16 / 7 + 2 / 5 ( 17/ 2 ) * [ 20/ 3

( 16/ 7 + 1 / 5 * 17/ 6 )*20 / 3

( 16/ 7 + 17/ 30 )*20/ 3

( 16* 30+ 17* 7 / 210 )*20 / 3

( 480 + 119 / 210 )*20/ 3 599 / 210 * 20 / 3

599 * 2 / 63

1198 / 69

=19 ^ 1 / 63

1 b )

Sin 48 =x / 250

X =250 sin 48 degrees

X = 250 * 0 . 7431

X =185 . 7775 m

=186 m

2 )

Let musa ‘ s age =x .

Manya ‘ s age =y .

x – y =3 – – – – – – – – – ( 1 )

Also x =3 + y – – – – – – ( 2 )

7 years ago

Musa ‘ s age =x – 7

Manya ‘ s age =y – 7

x – 7 =2 ( y – 7 )

x – 7 =2 y – 14

x – 2 y =- 14 + 7

x – 2 y =- 7 – – – – – – – eqn ( 3 )

Put eqn ( 2 ) into eqn( 3 )

3 + y – 2 y =- 7

– y = – 7 – 3

– y = – 10

Y =10

But x = 3 + 10 =====>x =13

Also therefore Musa ‘ s age is x =13 ,

And Manya’ s age is y =10

2 b )

Let the time be y

( x + y ) + ( x + 3 + y ) = 45

( 10 + y ) + ( 10 + 3 + y ) = 45

10 + 10 + 3 + 2 y = 45

23 + 2 y = 45

2 y = 45 – 23

2 y = 22

Y = 22 / 2

Y = 11 years

The sum of their ages will be 45 after 11 years

3 )

CIRCUMFERENCE OF TWO SEMI CIRCLES* =PIEd

22 / 7 X 120

= * 377 . 142 *

2 ( 377 . 142 + 60)

=874 . 29 Km

3 b )

Velocity = distance / time

Distance = 1474 . 28 m / 1000

=1 . 47km

Time = 200 s =200 / 60 * 60

=200 / 3600

Time = 0 . 05 hr

Velocity = 1 . 47 / 0 . 05 =29. 4 km/ hr